This article by the investor Claire Barnes of Apollo Investment Management highlights an interesting property of compound growth in any quantity which forms part of a finite whole. In summary, the point is this. Suppose urban land area + rural land area = 1 (ie a finite land area), and assume urban land area grows at a constant percentage rate (in line with the ‘economic growth’ universally sought by governments). Then it follows that rural land area doesn’t just decline, it declines at an accelerating percentage rate.
Claire’s illustration copied below shows the case where rural land is initially 85% of the total, urban land is 15%, and urban land grows at 5% per annum. On these parameters, the rural land is extinguished after about 38 years.

Note the alarming and invidious property of the second graph. For many years the percentage rate of decline in the rural part is very small, too small for most people to notice. Then suddenly the rate of decline speeds up and the remaining rural part disappears quickly, too quickly to do anything about it.
Algebraically, we can represent this as follows.
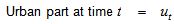
Assuming that the urban part grows a constant rate g per annum, we have
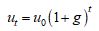
Now note that continuously compounded percentage rates of growth can also be expressed as semi-logarithmic derivatives, i.e. d (log x) / dt (this will be useful in a moment). So taking logs of the last equation above, we have

Also,
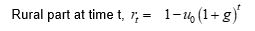
Then the continuously compounded percentage rate of decline of the rural part is the semi-logarithmic derivative d (log r) / dt. That is
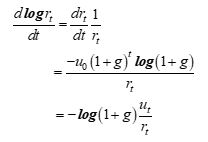
To interpret this, note that the urban part increases at a constant continuous rate log (1+g). The rural part decreases at an accelerating rate. The accelerating rate is log (1+g) scaled by the current ratio of the two parts.
For small g, log (1+g) ≈ g (e.g. log 1.05 = 0.0479), and so we can forget about taking logs and just scale the growth rate g by the current ratio of the two parts. This makes intuitive sense – in some sense the “same” process is affecting the rural part, it just has to be scaled for the continuously varying relative sizes of the two parts.
The time until the rural part is exhausted is given by setting the equation for “rural part at time t” to 0. This gives
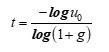
which for u(0) = 15% and g = 5% gives 38.9 years, consistent with the graph above. In words: the time to extinction of the rural part is inversely proportional to the growth rate of the urban part.
Pages 12-13 of this report from the World Bank give some data on current urban land and rates of growth, which suggest that for most Asian countries, the outlook is not quite as bad as suggested by the graph above. But there are probably ambiguities with the definition and measurement of “urban” and “rural”. And whatever the exact parameters, a precautionary principle seems sensible, because the overall pattern is quite general: when one part increases at a constant percentage rate, the other part doesn’t just decline, it declines at an accelerating percentage rate.
I agree that this does not seem to be widely appreciated. Perhaps it is appreciated by ecologists, but on a quick search I could not find obviously relevant commentary. Claire suggests a couple of reasons why it may be neglected. First, individuals and governments tend to focus more on things which show compound growth, because that is where his response investment and career and taxation opportunities tend to be found. We tend to be less aware of complementary things which are declining. Second, evidence-based people focus on things which are quantified; but things which are declining tend not to be quantified, precisely because of the lack of positive opportunities. I agree with both these points.
The alarming property of the graph is the “gradually, then suddenly” pattern of the rural part’s decline. This is not really exponential (a constant percentage rate of decline), it’s more alarming than that: it’s an accelerating percentage rate of decline. Following the famous quote about bankruptcy, perhaps we can call it Hemingway decline.
We can then summarise, loosely speaking, like this:
Where two parts form a finite whole, and one part increases at a constant percentage rate, the second part declines at an acccelerating percentage rate. It declines gradually, then suddenly: Hemingway decline